How to find oblique asymptotes using limits

We have shown how to use the first and second derivatives of a function to describe the shape of a graph. In this section, we define limits at infinity and show how these limits affect the graph of a function.

We use MathJax. Many functions exhibit asymptotic behavior. Graphically, that is to say that their graph approaches some other geometric object usually a line as the graph of the function heads away from the area around the origin. In other words, asymptotic behavior involves limits, since limits are how we mathematically describe situations where a function approaches a value. In college algebra, you may have learned how to locate several type of asymptotes. Calculus allows us to confirm these locations, by justifying their existence through limits. Therefore, to find horizontal asymptotes, we simply evaluate the limit of the function as it approaches infinity, and again as it approaches negative infinity.

How to find oblique asymptotes using limits

.

Consider a rational function. What is really required to justify this statement is either the Sandwich Theorem or a delta-epsilon proof or actually, an M-epsilon proof.

.

We have shown how to use the first and second derivatives of a function to describe the shape of a graph. In this section, we define limits at infinity and show how these limits affect the graph of a function. We begin by examining what it means for a function to have a finite limit at infinity. Then we study the idea of a function with an infinite limit at infinity. Back in Introduction to Functions and Graphs, we looked at vertical asymptotes; in this section we deal with horizontal and oblique asymptotes. We can extend this idea to limits at infinity.

How to find oblique asymptotes using limits

We have shown how to use the first and second derivatives of a function to describe the shape of a graph. In this section, we define limits at infinity and show how these limits affect the graph of a function. At the end of this section, we outline a strategy for graphing an arbitrary function f. We begin by examining what it means for a function to have a finite limit at infinity. Then we study the idea of a function with an infinite limit at infinity. Back in Introduction to Functions and Graphs , we looked at vertical asymptotes; in this section we deal with horizontal and oblique asymptotes. We can extend this idea to limits at infinity.

Fireman salary australia

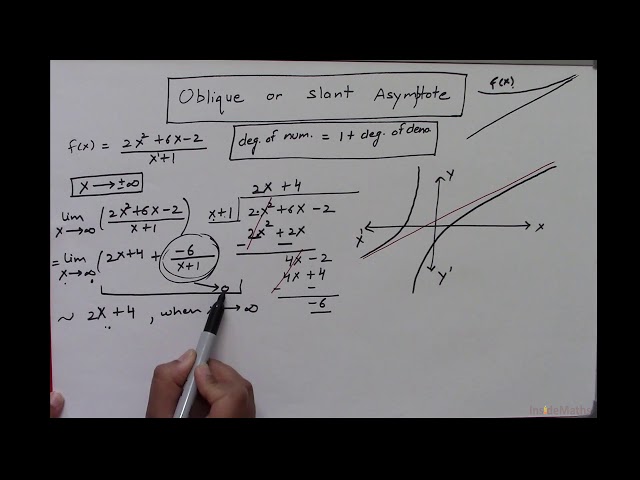
After doing so and applying algebraic limit laws, we obtain. In a rational function, the denominator cannot be zero. An oblique linear asymptote occurs when the graph of a function approaches a line that is neither horizontal nor vertical. Although these terms provide accurate descriptions of limits at infinity, they are not precise mathematically. Sign in. Recognize an oblique asymptote on the graph of a function. A function can have at most two oblique linear asymptotes. Here we use the formal definition of limit at infinity to prove this result rigorously. Consider a polynomial function. We then look at how to use these definitions to prove results involving limits at infinity. When doing so, we see that. To find possible locations for the vertical asymptotes, we check out the domain of the function. We say a function has a negative infinite limit at infinity and write. To find the oblique asymptote, use long division of polynomials to write. A handwaving argument of this style is typically used in this situation.

In my experience, students often hit a roadblock when they see the word asymptote. What is an asymptote anyway?

This is another handwaving argument, as we had in the previous example, and it also really requires either the Sandwich Theorem or a delta-epsilon proof. In fact, a function may cross a horizontal asymptote an unlimited number of times. Recognize an oblique asymptote on the graph of a function. Step 2 : Find the intercepts. If a rational function has an oblique linear asymptote, it can be found by division. A handwaving argument of this style is typically used in this situation. Since there is only one solution, there can be at most one vertical asymptote. Many functions exhibit asymptotic behavior. Determine the point on the graph where a cusp is located. We can extend this idea to limits at infinity. Then we study the idea of a function with an infinite limit at infinity. Graphically, that is to say that their graph approaches some other geometric object usually a line as the graph of the function heads away from the area around the origin. Formal Definitions Earlier, we used the terms arbitrarily close, arbitrarily large, and sufficiently large to define limits at infinity informally. Here we use the formal definition of limit at infinity to prove this result rigorously. Since the two limits were identical, this function has a single horizontal asymptote.