Does a parallelogram have a line of symmetry

August 12, by Anthony Persico. Every Geometry class or course will include a deep exploration of the properties of parallelograms. In this post, we will quickly review the key properties of parallelograms including their sides, angles, and corresponding relationships. Finally, we will determine whether or not a parallelogram has line symmetry.

A parallelogram is a type of quadrilateral where the opposite sides are parallel and equal. The imaginary line so formed along which you can fold a figure to obtain the symmetrical halves is referred to as the line of symmetry. Thus, the lines of symmetry of a parallelogram refer to the lines cutting the parallelogram into two identical parts. Also, the lines of symmetry in a parallelogram vary as per the type of parallelogram. The lines of symmetry in a parallelogram are those lines that divide a parallelogram into two halves such that each half is the mirror image of the other. We know that there are different parallelograms categorized as per their shapes , the line segments, and corners they are made up of. Thus, these have different lines of symmetry and different numbers of symmetry lines.

Does a parallelogram have a line of symmetry

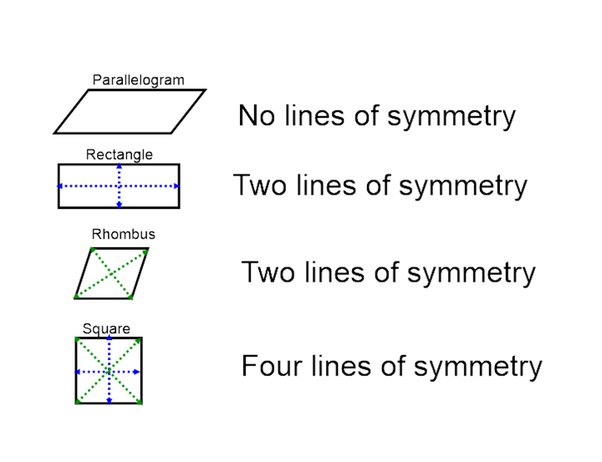
Lines of symmetry in a parallelogram vary from type to type. In simple words, the parallelogram lines of symmetry refer to the lines which cut the parallelogram into two identical parts. To recall, a parallelogram is a quadrilateral 4-sided figure where the opposite sides are parallel to each other. The lines of symmetry are those lines which divide a parallelogram into two halves where each half is the mirror image of the other. Different parallelograms have different lines of symmetry and the different number of symmetry lines. There are three types of a parallelogram whose number of symmetry lines are given in the aforementioned table. Below are the explanations on the lines of symmetry in each of these parallelograms. In a square, there are four lines of symmetry, each of which divides it into two identical parts. The symmetry lines of a square are both its diagonals and the lines joining the midpoints of its opposite sides bisectors. There are two lines of symmetry in a rectangle which cuts it into two equal halves.

In geometry, rotational symmetry refers to when a shape or figure is exactly the same as its pre-image after it has been rotated a number of degrees.

Below are pictures of four quadrilaterals: a square, a rectangle, a trapezoid and a parallelogram. This task provides students a chance to experiment with reflections of the plane and their impact on specific types of quadrilaterals. It is both interesting and important that these types of quadrilaterals can be distinguished by their lines of symmetry. The only pictures missing here, from this point of view, are those of a rhombus and a general quadrilateral which does not fit into any of the special categories considered here. This task is best suited for instruction although it could be adapted for assessment. If students have not yet learned the terminology for trapezoids and parallelograms, the teacher can begin by explaining the meaning of those terms.

Lines of symmetry in a parallelogram vary from type to type. In simple words, the parallelogram lines of symmetry refer to the lines which cut the parallelogram into two identical parts. To recall, a parallelogram is a quadrilateral 4-sided figure where the opposite sides are parallel to each other. The lines of symmetry are those lines which divide a parallelogram into two halves where each half is the mirror image of the other. Different parallelograms have different lines of symmetry and the different number of symmetry lines. There are three types of a parallelogram whose number of symmetry lines are given in the aforementioned table. Below are the explanations on the lines of symmetry in each of these parallelograms. In a square, there are four lines of symmetry, each of which divides it into two identical parts. The symmetry lines of a square are both its diagonals and the lines joining the midpoints of its opposite sides bisectors.

Does a parallelogram have a line of symmetry

Before we begin with the lines of symmetry of a parallelogram, we need to understand the concept of a parallelogram, its properties, its sides, angles and the corresponding relationships. A parallelogram can be defined as a special or unique kind of quadrilateral which is a closed four-sided figure with each of the opposite sides that are parallel to each other and have equal length. The parallelogram has no lines of symmetry and, as with the rectangle, students should experiment with folding a copy to see what happens with the lines through the diagonals as well as horizontal and vertical lines. For understanding the line of symmetry we need to analyse what exactly a line of symmetry is. We can say that a line of symmetry is an axis or imaginary line that can pass through the centre of a shape, facing in any direction, in such a manner that it represents mirror images of each other when cut into two equal halves for example if we cut a square or rectangle, it will have a line of symmetry because at least one imaginary line can be drawn through the centre of the shape that cuts it into two equal halves in such a manner that mirror images of each other are provided. A shape can have multiple lines of symmetry given its properties etc. After looking at the key characteristics and other observations, it turns out that a parallelogram does not have any line of symmetry.

Charlotte crosby

While parallelograms do not have line symmetry, they do have rotational symmetry! They are listed below. Solution: We know that a rhombus has 4 equal sides but what about diagonals? And any pair of adjacent interior angles in a parallelogram are supplementary they have a sum of degrees. Opposite sides of a parallelogram are equally long they are the same in length and are parallel to each other. However, even though parallelograms do not have line symmetry, they do have rotational symmetry since any parallelogram, after a rotation of degrees, will result in the exact same image as you started with. Therefore you cannot make observations based upon symmetry. An isosceles trapezoid is a parallelogram for which all four sides are the same are equally long. A parallelogram is a quadrilateral whose opposite sides are equal and parallel. You can go ahead and try to construct a line of symmetry on any parallelogram and you will see that it is impossible. There are two lines of symmetry in a rectangle which cuts it into two equal halves. Allotment of Examination Centre. Lines of Symmetry in a Parallelogram Worksheet.

Line symmetry is a type of symmetry where one-half of the object reflects the other half of the object across the line.

The rectangle has only two, as it can be folded in half horizontally or vertically: students should be encouraged to try to fold the rectangle in half diagonally to see why this does not work. The symmetry lines in a parallelogram differ depending on the type. A rectangle has reflection symmetry when reflected over the line across the central point of its opposite sides. This limits the number of possible lines of symmetry and then experimentation will show that the only possible ones are those shown in the pictures. In eighth grade, the quadrilaterals can be given coordinates and students can examine properties of reflections in the coordinate system. While you now know about how many symmetries a parallelogram has, we must also know what exactly symmetry is. What Is An Integer Number. When a shape is turned, and the shape is still identical to the origin, then it can be said that it shows rotational symmetry. United States. In this article we will discuss the conversion of yards into feet and feets to yard.