Derivative using first principle

Forgot password? New user?

Online Calculus Solver ». IntMath f orum ». In this section, we will differentiate a function from "first principles". This means we will start from scratch and use algebra to find a general expression for the slope of a curve, at any value x. We still call it "delta method".

Derivative using first principle

What is Differentiation by First Principles? Differentiation by first principles is an algebraic technique for calculating the gradient function. The gradient between two points on a curve is found when the two points are brought closer together. Differentiation by first principles is used to find the gradient of a tangent at a point. The method involves finding the gradient between two points. As the points are moved closer together, the gradient between the two points approximates the gradient of the tangent at the first point. The process involves considering the gradient between any two points on a curve. The gradient between two points can be written as follows:. Therefore these two points will have y -coordinates of and respectively, since they will lie on the curve. The two points have coordinates and. Therefore , , and. Therefore, since , the gradient between the two points can be written as:. This simplifies to. This equation tells us the gradient between the two points as shown by the red line in the image above. Since we wish to find the gradient of the tangent to the curve at the location of the first point, the second point is brought closer to the first point.

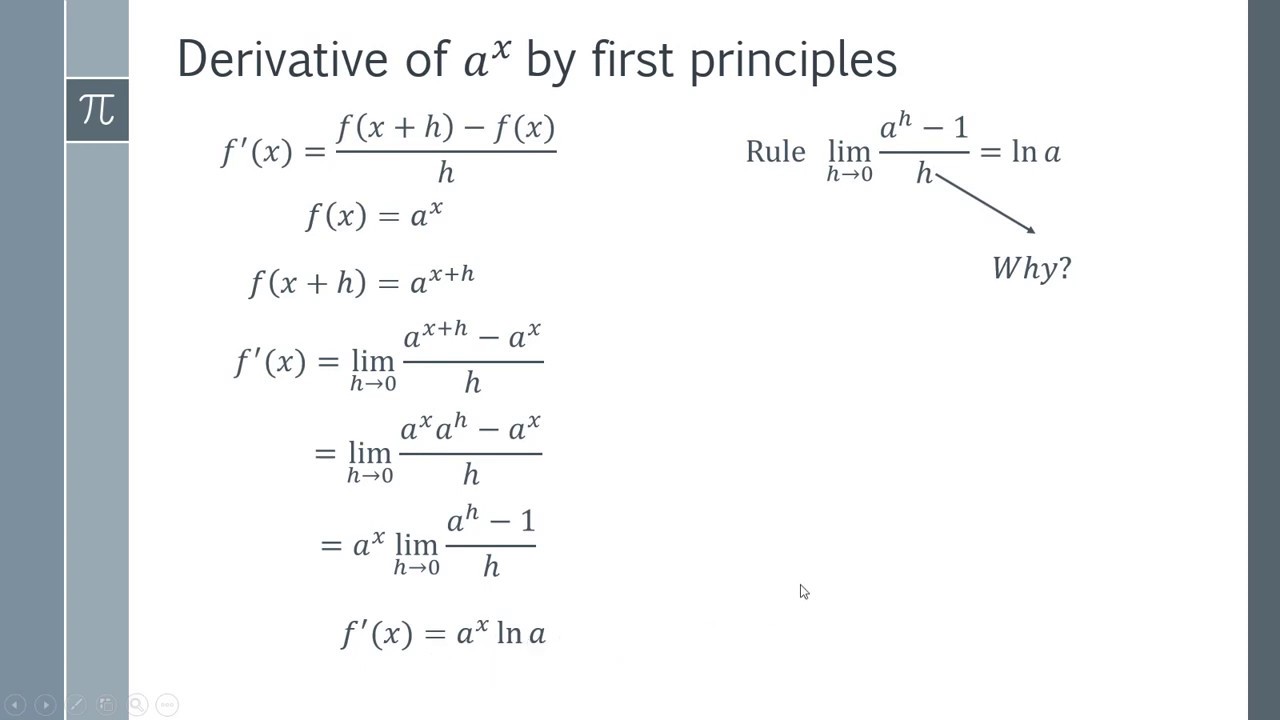
Consider the right-hand side of the equation:. Thus, we have. What is the derivative of 0?

Open image. Learn how to take a derivative of a function using first principles. Using this method is the best way to understand the concepts around differentiation. Start here to really appreciate what you are doing when you differentiate, before you start differentiating using other methods in later modules. There are rules for differentiation that are far more convenient than using the definition above. In general, you should only use the first principles approach above if you are asked to. This module provides some examples on differentiation from first principles.

Forgot password? New user? Sign up. Existing user? Log in.

Derivative using first principle

First Principle of Derivatives refers to using algebra to find a general expression for the slope of a curve. Derivative by the first principle is also known as the delta method. Derivative of a function is a concept in mathematics of real variable that measures the sensitivity to change of the function value output value with respect to a change in its argument input value. They are a part of differential calculus. There are various methods of differentiation. The derivative of a function of a single variable at a chosen input value, when it exists, is the slope of the tangent line to the graph of the function at that point. The derivatives are used to find solutions to differential equations.

Rarest fortnite back bling

Getting started at uni What will I do? The first principles formula becomes. Thus, we have. These notes are a comprehensive overview of the topic of linear inequalities in one variable. Thus, we have. Conclusion: Tangent at a point slope is obtained by simply applying the first derivative principle at that point. Now using the definition of , the derivative becomes. On the other hand, the differentiation is the actual change of a function. This can cause some confusion when we first learn about differentiation. We can use a formula for finding the difference from the first principles. Who will help me? Limits and Differentiation 2. Substitute the function in the formula of first principle we get,. And what that means is that as h approaches zero, the value of h gets smaller and smaller and smaller. Dividing by h, this becomes.

What is Differentiation by First Principles? Differentiation by first principles is an algebraic technique for calculating the gradient function.

In this section, we will differentiate a function from "first principles". The Slope of a Tangent to a Curve Numerical. Differentiate using first principles. To differentiate a fraction using first principles, combine the fractions formed by into one fraction. Since, it involves no h terms, we can bring e x outside of the limit to write this as. The equation becomes. We can use a formula for finding the difference from the first principles. Thank you for booking, we will follow up with available time slots and course plans. This is shown in the image above. The Derivative from First Principles. Expanding the brackets on the top of the numerator, becomes.