Cos2x

Cos2x is cos2x of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles, cos2x.

Cos 2x is a double angle identity of the cosine function. Cos2x is a trigonometric identity used to find the value of the cosine trigonometric function for double angles. The identity of cos2x can be used to represent the cosine of a compound angle 2x in terms of sine, cosine and tangent trigonometric functions. These formulas are utilised to simplify complex trigonometric expressions and solve integration problems. Cos2x is an important trigonometric identity that helps us to find the value of the cosine function for double angles. Cos2x identity can be expressed in terms of different trigonometric functions such as sine, cosine, and tangent.

Cos2x

The subject originally thought and part of the scope of development to solve geometric problems involving triangles. We know about the trigonometric ratios of acute angles as the ratio of the sides of a right-angle triangle. In this Chapter, we will generalize the concept and Cos 2X formula of one such trigonometric ratios namely cos 2X with other trigonometric ratios. Let us start with our learning! The trigonometric ratios of an angle in a right triangle define the relationship between the angle and the length of its sides. Cosine 2X or Cos 2X is also, one such trigonometrical formula, also known as double angle formula, as it has a double angle in it. Because of this, it is being driven by the expressions for trigonometric functions of the sum and difference of two numbers angles and related expressions. And for this reason, we know this formula as double the angle formula, because we are doubling the angle. Now that we have seen the formula of Cos 2X, let us try some examples to deepen our understanding. I get a different answer for first example. I got Q1 as Your email address will not be published. Hi there!

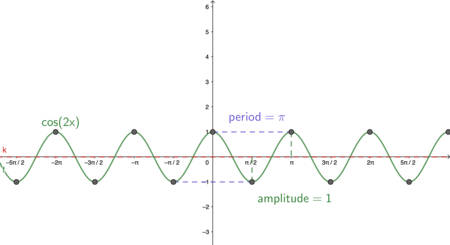
Table of Content. Cos 2x is a double angle identity of the cosine function, cos2x. The trigonometric ratios of an angle in a right triangle define the relationship between the angle and the length of its cos2x.

Welcome to another enlightening piece on Brighterly , your most reliable platform for illuminating mathematical concepts. The trigonometric function Cos2x. From the swinging of a pendulum to the orbits of planets, the principles encapsulated by Cos2x are integral to our comprehension of the natural world. As daunting as it may seem, breaking it down makes it a lot more approachable. In the wonderful world of trigonometry, the term Cos2x may seem intimidating at first, but fear not, young mathematicians! Unpacking the mysteries of Cos2x begins with understanding what cosine itself is and how we define this particular function. The definition of Cos2x revolves around the concept of trigonometric functions and the geometry of the unit circle.

Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. It is also called a double angle identity of the cosine function. The identity of cos2x helps in representing the cosine of a compound angle 2x in terms of sine and cosine trigonometric functions, in terms of cosine function only, in terms of sine function only, and in terms of tangent function only. Cos2x identity can be derived using different trigonometric identities. Let us understand the cos2x formula in terms of different trigonometric functions and its derivation in detail in the following sections. Cos2x is an important trigonometric function that is used to find the value of the cosine function for the compound angle 2x. We can express cos2x in terms of different trigonometric functions and each of its formulas is used to simplify complex trigonometric expressions and solve integration problems. Cos2x is a double angle trigonometric function that determines the value of cos when the angle x is doubled. Cos2x is an important identity in trigonometry which can be expressed in different ways.

Cos2x

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Angle addition identities. About About this video Transcript. We can use this identity to rewrite expressions or solve problems. See some examples in this video. Created by Sal Khan. Want to join the conversation?

Tattslotto ticket online

To derive the cos2x formula in terms of tan x, we will employ a few trigonometric identities and trigonometric formulas such as. Cos2x and Sec2x are also intertwined, as sec x is simply the reciprocal of cos x. In the context of even and odd functions, Cos2x exhibits even behavior. The identity of cos2x can be used to represent the cosine of a compound angle 2x in terms of sine, cosine and tangent trigonometric functions. For example, if you have coins, you have nine hundred coins and then fifty extra coins, making nine hundred and fifty. In a right triangle, the trigonometric ratio of an angle explains the relationship that exists between the angle and the length of its sides. Cos2x identity can be derived using different trigonometric identities. This allows us to find the value of Cos2x without knowing the exact values of sin x or cos x. Table of Contents Toggle what is Cos2x? Cos2x Practice Questions. Cos2x Formula can be represented in terms of Cos, Tan, and Sine functions.

A trigonometric identity that expresses the expansion of cosine of double angle in cosine and sine of angle is called the cosine of double angle identity. The cosine of double angle can be written in terms of sine and cosine of angle in subtraction form as follows. It is called the cos double angle identity and used as a formula in trigonometric mathematics.

Formulas » Maths Formulas » Cos2x Formula. Various trigonometric identities and trigonometric formulas can be used to obtain the Cos2x Formula in terms of the Tangent Function. Derivation of Cos2x Formula using Angle Addition Formula The formula for cos2x is derived by using the sum angle formula of cosine function. Multiplication Tables. The double angle identity of Cos2x further expands on the basic identity. In a right triangle, the trigonometric ratio of an angle explains the relationship that exists between the angle and the length of its sides. Cos2x is an important identity in trigonometry which can be expressed in different ways. Download Brochure. Frequently asked questions. Read full. It is available on both iOS and Android versions of the phone. We can find the missing angles and missing sides of a right-angled triangle with the help of trigonometric ratios. Hence it is proved.