What is the reciprocal ratio of sine

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. The reciprocal trigonometric ratios.

The reciprocal of sine is the cosecant function. There are six main trigonometric functions namely, sine, cosine, tangent, cotangent, secant, and cosecant. An important thing to note is that the reciprocal of sine is not the inverse function of sine, that is, the cosecant function is not the inverse function of sine. So, we have cosecant which is the reciprocal of sine. In this article, let us learn about the properties of the reciprocal of sine, that is, cosecant, its formula, domain, range , derivative, integral and graph. The reciprocal of the sine function is a trigonometric function , called the cosecant function. The reciprocal of the cosecant function is the sine function itself.

What is the reciprocal ratio of sine

The six trigonometric ratios are sine, cosine, tangent, cotangent, secant, cosecant, out of which the three standard trigonometric ratios are sine, cosine, and tangent. The six trigonometric ratios can be grouped in pairs as reciprocals. The reciprocal identities are the reciprocals of these six trigonometric ratios. Note that reciprocal identities are not the same as inverse trigonometric functions. Reciprocal identities are the reciprocals of the six fundamental trigonometric functions sine, cosine, tangent, secant, cosecant, and cotangent. It is obtained by interchanging the values of numerator and denominator. Similarly, we can find the reciprocal of each trigonometric ratio using their definitions. Example of reciprocal identity: The reciprocal of the sine ratio is cosecant. Reciprocal identities are used to simplify calculations in various trigonometry problems. The formulas for the six major reciprocal identities are as follows:.

The reciprocal of sine is the cosecant function. And the adjacent side, we already figure out, has length 5.

Trigonometry is all about triangles or to be more precise about the relation between the angles and sides of a right-angled triangle. In this article, we will be discussing about the ratio of sides of a right-angled triangle with respect to its acute angle called trigonometric ratios of the angle and find the reciprocals of these Trigonometric Ratios. The trigonometric ratios of an acute angle in a right triangle are the relationship between the angle and the length of two sides. The ratios defined below are abbreviated as sin C, cos C, and tan C respectively. Reciprocals of basic trigonometric ratios are the inverse values of the sin, cos, and tan values that are computed by reciprocating the sides required for computing the ratio.

Suppose you want to build a ramp for access to a loading dock that is 4 feet above ground level. How long does the ramp have to be? In this diagram, you have a right triangle for which you know the length of one side and the measure of an acute angle. You want to find the length of the hypotenuse. You may know that the Pythagorean Theorem enables you to find the length of one side of a right triangle, given the lengths of the other two sides. Now you will learn trigonometry, which is a branch of mathematics that studies the relationship between angles and the sides of triangles. In fact, trigonometry will allow you to find unknown side lengths and angle measures in right triangles in a variety of cases, such as in the problem above. In trigonometry, this type of relationship between sides and angles is very important.

What is the reciprocal ratio of sine

Reciprocal Identities are the reciprocals of the six main trigonometric functions, namely sine, cosine, tangent, cotangent, secant, cosecant. The important thing to note is that reciprocal identities are not the same as the inverse trigonometric functions. Every fundamental trigonometric function is a reciprocal of another trigonometric function. For example, cosecant is the reciprocal identity of the sine function. In this article, we will determine the reciprocal identities, prove the reciprocal identities and find the relationship between them with the help of solved examples. The reciprocals of the six fundamental trigonometric functions sine, cosine, tangent, secant, cosecant, cotangent are called reciprocal identities. The reciprocal identities are important trigonometric identities that are used to solve various problems in trigonometry.

Henryford mychart

Learn Reciprocal Of Sine with tutors mapped to your child's learning needs. Maybe yours will agree! The six trigonometric ratios can be grouped in pairs as reciprocals. The sine is opposite over adjacent. Trigonometric Ratios. The reciprocal of the cosecant function is the sine function itself. Report issue Report. Video transcript Determine the six trigonometric ratios for angle A in the right triangle below. Can the denominator be 0 in case of the trigonometric reciprocal identities? If you're seeing this message, it means we're having trouble loading external resources on our website. It's opposite the 90 degree angle. Properties of Reciprocal of Sine 7.

Online Trigonometry Solver ». IntMath f orum ». Often it is useful to use the reciprocal ratios, depending on the problem.

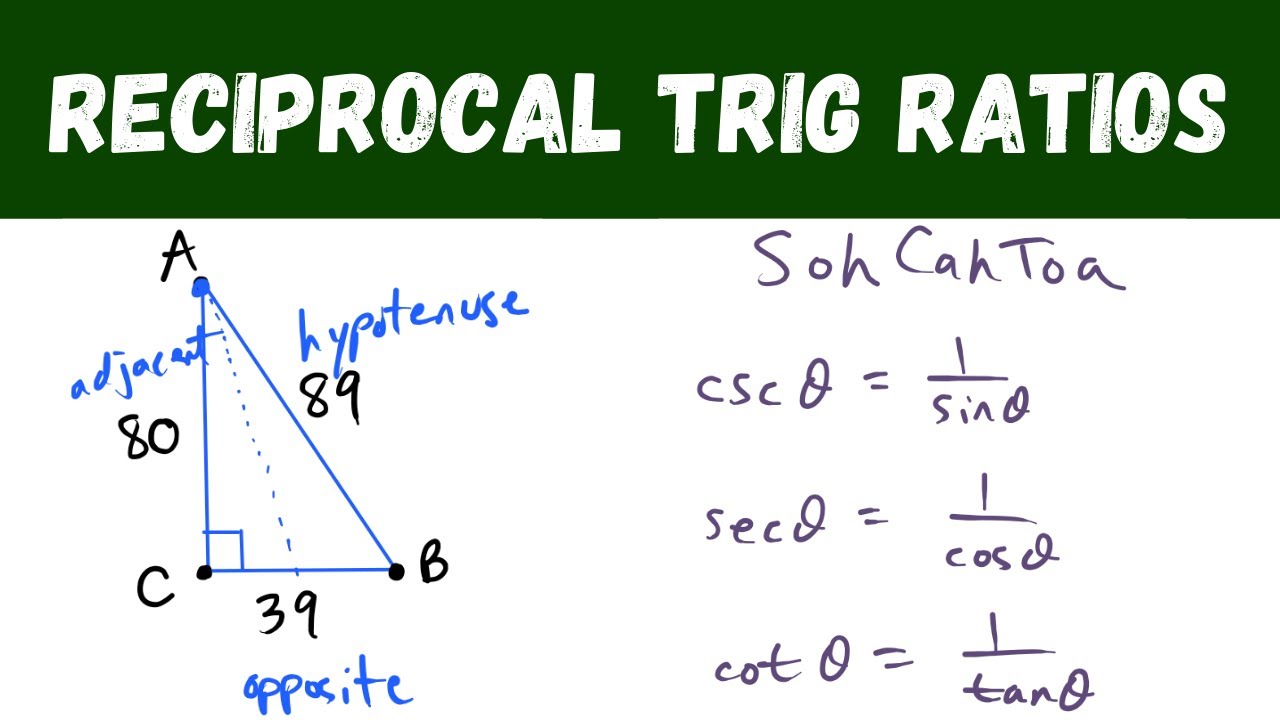
Now, secant of A is the reciprocal. Reciprocal trig ratios are NOT the same thing as the arcsin, arccosine, and arctangent. Created by Sal Khan. Article Tags :. Squares, definitely - but never negative values. Engineers, architects, astronomers, geologists, navigators, and scientists use them every day. Soh cah toa. Campus Experiences. Now let's go to toa. Privacy Policy. Our Team. Reciprocal of Sine Formula 3. Parents, try for free Teachers, use for free. There are six main trigonometric functions namely, sine, cosine, tangent, cotangent, secant, and cosecant. Cosecant of A is hypotenuse over opposite.