Solve bvp

Before we start off this solve bvp we need to solve bvp it very clear that we are only going to scratch the surface of the topic of boundary value problems. There is enough material in the topic of boundary value problems that we could devote a whole class to it. The intent of this section is to give a brief and we mean very brief look at the idea of boundary value problems and to give enough information to allow us to do some basic partial differential equations in the next chapter, solve bvp.

Help Center Help Center. This example shows how to use bvp4c to solve a boundary value problem with an unknown parameter. However, this only determines y x up to a constant multiple, so a third condition is required to specify a particular solution,. You can either include the required functions as local functions at the end of a file as done here , or save them as separate, named files in a directory on the MATLAB path. Create a function to code the equations. Note: All functions are included as local functions at the end of the example. Now, write a function that returns the residual value of the boundary conditions at the boundary points.

Solve bvp

The shooting method works by considering the boundary conditions as a multivariate function of initial conditions at some point, reducing the boundary value problem to finding the initial conditions that give a root. The advantage of the shooting method is that it takes advantage of the speed and adaptivity of methods for initial value problems. The disadvantage of the method is that it is not as robust as finite difference or collocation methods: some initial value problems with growing modes are inherently unstable even though the BVP itself may be quite well posed and stable. The shooting method looks for initial conditions so that. Since you are varying the initial conditions, it makes sense to think of as a function of them, so shooting can be thought of as finding such that. After setting up the function for , the problem is effectively passed to FindRoot to find the initial conditions giving the root. The default method is to use Newton's method, which involves computing the Jacobian. While the Jacobian can be computed using finite differences, the sensitivity of solutions of an initial value problem IVP to its initial conditions may be too much to get reasonably accurate derivative values, so it is advantageous to compute the Jacobian as a solution to ODEs. Then, differentiating both the IVP and boundary conditions with respect to gives. Since is linear, when thought of as a function of , you have , so the value of for which satisfies.

Using 5 and replacingand thinking of in terms of the other components ofyou get the nonlinear equation.

Help Center Help Center. This example uses bvp4c with two different initial guesses to find both solutions to a BVP problem. You either can include the required functions as local functions at the end of a file as done here , or save them as separate, named files in a directory on the MATLAB path. Create a function to code the equation. These inputs are automatically passed to the function by the solver, but the variable names determine how you code the equations. In this case, you can rewrite the second-order equation as a system of first-order equations.

The pycse book. The pycse blog. Adapted from Example 8. This is a boundary value problem not an initial value problem. First we consider using a finite difference method. We discretize the region and approximate the derivatives as:. The set of equations to solve is:.

Solve bvp

Adapted from Example 8. This is a boundary value problem not an initial value problem. First we consider using a finite difference method. We discretize the region and approximate the derivatives as:. The set of equations to solve is:. Since we use a nonlinear solver, we will have to provide an initial guess to the solution. We will in this case assume a line.

English up 6 students book cevap anahtarı

Here is a boundary value problem that does not have a unique solution. Now, write a function that returns the residual value of the boundary conditions at the boundary points. NDSolve issues a warning message because the matrix to solve for the initial conditions is singular, but has a solution:. To increase the likelihood that the computed eigenfunction corresponds to the fourth eigenvalue, you should choose an initial guess that has the correct qualitative behavior. These residual values are enforced at the first and last points of the mesh that you specify to bvpinit in your initial guess. The one exception to this still solved this differential equation except it was not a homogeneous differential equation and so we were still solving this basic differential equation in some manner. We will, on occasion, look at some different boundary conditions but the differential equation will always be on that can be written in this form. There is an alternative, nonlinear way to set up the auxiliary problems that is closer to the original method proposed by Gelfand and Lokutsiyevskii. This will be a major idea in the next section. Do you want to open this example with your edits? Toggle Main Navigation. Shooting from , the "Shooting" method gives warning messages about an ill-conditioned matrix and that the boundary conditions are not satisfied as well as they should be. The idea is to establish a set of auxiliary problems that can be solved to find initial conditions at one of the boundaries.

The shooting method works by considering the boundary conditions as a multivariate function of initial conditions at some point, reducing the boundary value problem to finding the initial conditions that give a root.

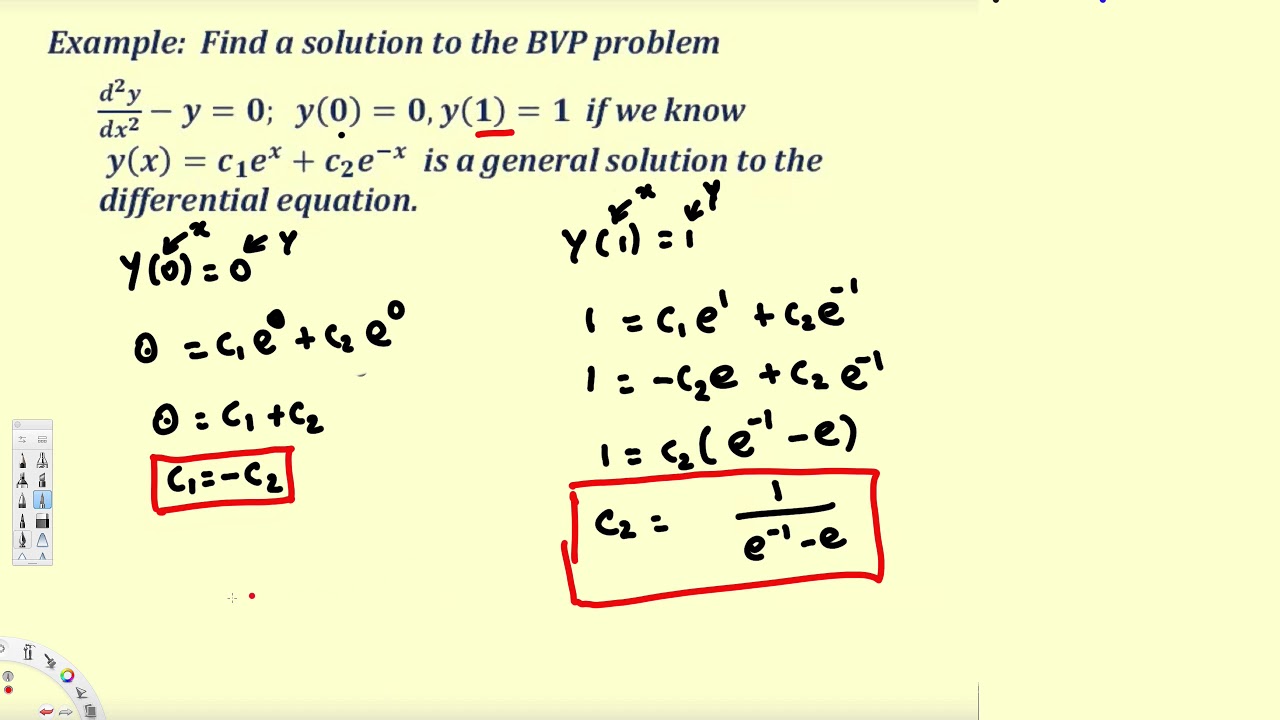
Due to the nature of the mathematics on this site it is best views in landscape mode. Example 5 Solve the following BVP. Differentiating the equation for gives. While the Jacobian can be computed using finite differences, the sensitivity of solutions of an initial value problem IVP to its initial conditions may be too much to get reasonably accurate derivative values, so it is advantageous to compute the Jacobian as a solution to ODEs. The method of chasing came from a manuscript of Gelfand and Lokutsiyevskii first published in English in [ BZ65 ] and further described in [ Na79 ]. The results are then combined into the matrix of 3 that is solved for to obtain the initial value problem that NDSolve integrates to give the returned solution. It does however exhibit all of the behavior that we wanted to talk about here and has the added bonus of being very easy to solve. Fourth eigenvalue is approximately The chasing method amounts to finding a vector function such that and. The mesh for x does not need to have a lot of points, but the first point must be 0. Based on your location, we recommend that you select:. There is another important reason for looking at this differential equation. Notes Quick Nav Download. No, overwrite the modified version Yes.