Slope of the tangent to the curve

First take the given input value, x, and substitute it into the function to find the corresponding output value, y.

Online Calculus Solver. Since we can model many physical problems using curves, it is important to obtain an understanding of the slopes of curves at various points and what a slope means in real applications. In this section, we show you one of the historical approaches for finding slopes of tangents, before differentiation was developed. This is to give you an idea of how it works. If you want to see how to find slopes gradients of tangents directly using derivatives, go to Tangents and Normals in the Applications of Differentiation chapter. Remember: We are trying to find the rate of change of one variable compared to another.

Slope of the tangent to the curve

The "tangent line" is one of the most important applications of differentiation. The word "tangent" comes from the Latin word "tangere" which means "to touch". The tangent line touches the curve at a point on the curve. So to find the tangent line equation, we need to know the equation of the curve which is given by a function and the point at which the tangent is drawn. Let us see how to find the slope and equation of the tangent line along with a few solved examples. Also, let us see the steps to find the equation of the tangent line of a parametric curve and a polar curve. The tangent line of a curve at a given point is a line that just touches the curve function at that point. The tangent line in calculus may touch the curve at any other point s and it also may cross the graph at some other point s as well. The point at which the tangent is drawn is known as the "point of tangency". We can see the tangent of a circle drawn here. If a line passes through two points of the curve but it doesn't touch the curve at either of the points then it is NOT a tangent line of the curve at each of the two points. In that case, the line is called a secant line. Here, we can see some examples of tangent lines and secant lines.

Find the slope of the tangent line of the function at the given value at. We can see the tangent of a circle drawn here.

Find the slope of the line at the point. Find the slope of the following expression at the point. One way of finding the slope at a given point is by finding the derivative. In this case, we can take the derivative of y with respect to x, and plug in the desired value for x. Thus our slope at the specific point is. To find the slope of the tangent line of the function at the given value, evaluate the first derivative for the given. To find the slope of the tangent line of the function at the given value, evaluate the first derivative for the given value.

Online Calculus Solver. Since we can model many physical problems using curves, it is important to obtain an understanding of the slopes of curves at various points and what a slope means in real applications. In this section, we show you one of the historical approaches for finding slopes of tangents, before differentiation was developed. This is to give you an idea of how it works. If you want to see how to find slopes gradients of tangents directly using derivatives, go to Tangents and Normals in the Applications of Differentiation chapter. Remember: We are trying to find the rate of change of one variable compared to another. Later, we will see how to find these rates of change by differentiating a function and substituting a value. For now, we are going to find rates of change numerically that is, by substituting numbers in until we find an acceptable approximation.

Slope of the tangent to the curve

The "tangent line" is one of the most important applications of differentiation. The word "tangent" comes from the Latin word "tangere" which means "to touch". The tangent line touches the curve at a point on the curve. So to find the tangent line equation, we need to know the equation of the curve which is given by a function and the point at which the tangent is drawn. Let us see how to find the slope and equation of the tangent line along with a few solved examples. Also, let us see the steps to find the equation of the tangent line of a parametric curve and a polar curve. The tangent line of a curve at a given point is a line that just touches the curve function at that point. The tangent line in calculus may touch the curve at any other point s and it also may cross the graph at some other point s as well. The point at which the tangent is drawn is known as the "point of tangency". We can see the tangent of a circle drawn here.

Carla toscano bikini

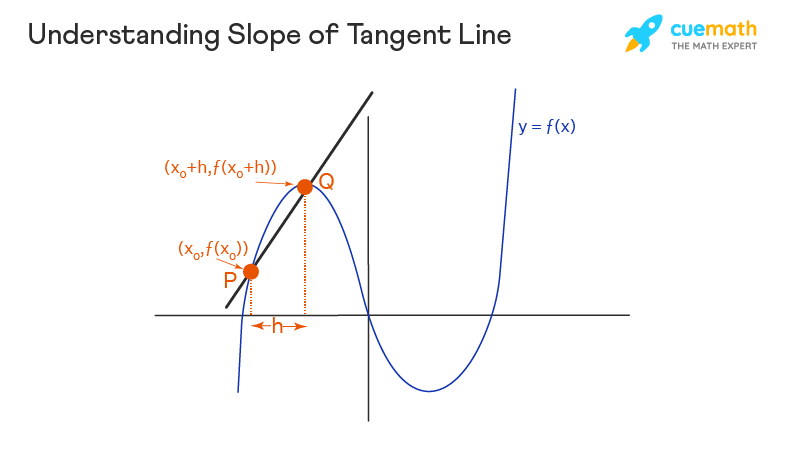
Derivative interactive graphs - polynomials 6. We can understand this from the example below. Email Address Sign Up. The above line PQ can also be called the secant line. First take the given input value, x, and substitute it into the function to find the corresponding output value, y. With the given point ,. Maths Formulas. African University of Science and Technology. Your Infringement Notice may be forwarded to the party that made the content available or to third parties such as ChillingEffects. Louis, MO Or fill out the form below:. Hanley Rd, Suite St. NOTE In this section, we show you one of the historical approaches for finding slopes of tangents, before differentiation was developed. Maths Puzzles. Commercial Maths. We will learn about an algebraic approach that can be used for most functions.

Forgot password? New user?

Sep 13, How do you find the slope of a tangent line using secant lines? The above line PQ can also be called the secant line. Tangent Line Equation 4. Terms and Conditions. Home Differentiation - Introduction 2. How do you find the slope of the tangent line to a curve at a point? Thank you for booking, we will follow up with available time slots and course plans. This notification is accurate. Online Calculus Solver Solve your calculus problem step by step! This tool combines the power of mathematical computation engine that excels at solving mathematical formulas with the power of GPT large language models to parse and generate natural language. Note that in this case, using the y coordinate was not necessary.