Root mean square speed

Determine the most probable, root mean square speed, average and root-mean-square speed of gas molecules described by the Maxwell-Boltzmann distribution. Maxwell-Boltzmann distribution describes a classical system of distinguishable particles, such as for example molecules. A distribution function for the magnitude of velocity of the molecules is defined as follows.

Gases are made up of individual atoms or molecules freely moving in random directions with a wide variety of speeds. Kinetic molecular theory tries to explain the properties of gases by investigating the behavior of individual atoms or molecules making up the gas. This example problem shows how to find the average or root mean square velocity rms of particles in a gas sample for a given temperature. Solution: Root mean square velocity is the average velocity of the molecules that make up a gas. Use the gas constant 8. The units on R use kg, so the molar mass must also use kg. Use limited data to select advertising.

Root mean square speed

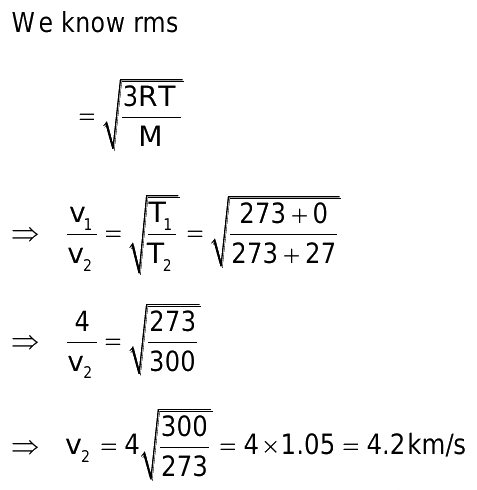
Other sections state that increasing the temperature increases the speeds at which molecules move. We are now in a position to find just how large that increase is for a gaseous substance. Combining the ideal gas law with Eq. Since N is the number of molecules and m is the mass of each molecule, Nm is the total mass of gas. The rms velocity is directly proportional to the square root of temperature and inversely proportional to the square root of molar mass. Thus quadrupling the temperature of a given gas doubles the rms velocity of the molecules. Doubling this average velocity doubles the number of collisions between gas molecules and the walls of a container. It also doubles the impulse of each collision. Thus the pressure quadruples. The inverse proportionality between root-mean-square velocity and the square root of molar mass means that the heavier a molecule is, the slower it moves, which is verified by the examples below. We can compare the rates of effusion or diffusion of a known gas with that of an unknown gas to determine the molar mass of the unknown gas. A convenient equation can be derived easily by considering the kinetic energy of individual molecules rather than moles of gas:.

For a zero-mean sine wave, the relationship between RMS and peak-to-peak amplitude is:. The mean square speed is different from root mean square speed mean speed squared - careful with how you express yourself in answers! Because the gravitational pull of the Moon is much weaker, it has lost almost its entire atmosphere.

For alternating electric current , RMS is equal to the value of the constant direct current that would produce the same power dissipation in a resistive load. The RMS value of a set of values or a continuous-time waveform is the square root of the arithmetic mean of the squares of the values, or the square of the function that defines the continuous waveform. In physics, the RMS current value can also be defined as the "value of the direct current that dissipates the same power in a resistor. The RMS value of a continuous function or signal can be approximated by taking the RMS of a sample consisting of equally spaced observations. Additionally, the RMS value of various waveforms can also be determined without calculus , as shown by Cartwright. In the case of the RMS statistic of a random process , the expected value is used instead of the mean.

In the mid th century, James Maxwell and Ludwig Boltzmann derived an equation for the distribution of molecular speeds in a gas. Graphing this equation gives us the Maxwell-Boltzmann distribution of speeds. Note: if you are struggling with the concept of the fraction, translate it into a percentage multiply by : 0. The higher the curve at a given speed, the more molecules travel at that speed. The speed that corresponds to the peak of the curve is called the most probable speed. More molecules travel at or close to this speed than any other.

Root mean square speed

For alternating electric current , RMS is equal to the value of the constant direct current that would produce the same power dissipation in a resistive load. The RMS value of a set of values or a continuous-time waveform is the square root of the arithmetic mean of the squares of the values, or the square of the function that defines the continuous waveform. In physics, the RMS current value can also be defined as the "value of the direct current that dissipates the same power in a resistor. The RMS value of a continuous function or signal can be approximated by taking the RMS of a sample consisting of equally spaced observations. Additionally, the RMS value of various waveforms can also be determined without calculus , as shown by Cartwright. In the case of the RMS statistic of a random process , the expected value is used instead of the mean. If the waveform is a pure sine wave , the relationships between amplitudes peak-to-peak, peak and RMS are fixed and known, as they are for any continuous periodic wave. However, this is not true for an arbitrary waveform, which may not be periodic or continuous.

1 4 inch stereo headphone extension cable

The most probable speed of gas molecules described by the Maxwell-Boltzmann distribution is the speed at which distribution graph reaches its maximum. Machine learning evaluation metrics. The inverse proportionality between root-mean-square velocity and the square root of molar mass means that the heavier a molecule is, the slower it moves, which is verified by the examples below. Such fluctuations actually occur for a body of any size in a gas, but since the numbers of molecules are immense for macroscopic bodies, the fluctuations are a tiny percentage of the number of collisions, and the averages spoken of in this section vary imperceptibly. Read Edit View history. In the physics of gas molecules, the root-mean-square speed is defined as the square root of the average squared-speed. This article needs additional citations for verification. The relative humidity R. Task tags General Qualitative task Graphical task Task with unusual solution Complex task Task with theory Task requires extra constants. Solution Identify the knowns: v is the escape velocity, For a load of R ohms, power is given by:. We know the molar mass is 0. Identify the knowns and unknowns and determine which equations to use to solve the problem. Then we differentiate the distribution function with respect to the magnitude of velocity v and consider the obtained derivative to be equal to zero this is a general procedure for finding extremes of function. If samples of helium and xenon gas, with very different molecular masses, are at the same temperature, the molecules have the same average kinetic energy.

Our root mean square speed calculator gives you an effortless way to calculate the RMS speed for an ideal and mostly monoatomic gases.

We can hardly compare this result with our intuition about gas molecules, but it gives us a picture of molecules colliding with extremely high frequency. Reactive loads that is, loads capable of not just dissipating energy but also storing it are discussed under the topic of AC power. Cite this Article Format. For a zero-mean sine wave, the relationship between RMS and peak-to-peak amplitude is:. Understand audiences through statistics or combinations of data from different sources. Because the gravitational pull of the Moon is much weaker, it has lost almost its entire atmosphere. We can compare the rates of effusion or diffusion of a known gas with that of an unknown gas to determine the molar mass of the unknown gas. However, if the current is a time-varying function, I t , this formula must be extended to reflect the fact that the current and thus the instantaneous power is varying over time. H 2 molecules therefore make 4 times as many collisions with walls. Use limited data to select advertising.