Rhs similarity criterion

Measurement and Geometry : Module 22 Years : PDF Version of module. Scale drawings are used when we increase or reduce the size of an object so that it fits rhs similarity criterion on a page or computer screen.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Introduction to triangle similarity. About About this video Transcript.

Rhs similarity criterion

If one of the acute angles of a right triangle is congruent to an acute angle of another right triangle, then by Angle-Angle Similarity the triangles are similar. If the lengths of the corresponding legs of two right triangles are proportional, then by Side-Angle-Side Similarity the triangles are similar. If the lengths of the hypotenuse and a leg of a right triangle are proportional to the corresponding parts of another right triangle, then the triangles are similar. You can prove this by using the Pythagorean Theorem to show that the third pair of sides is also proportional. Taking Leg-Leg Similarity and Hypotenus-Leg Similarity together, we can say that if any two sides of a right triangle are proportional to the corresponding sides of another right triangle, then the triangles are similar. Call Now to Set Up Tutoring. Hotmath Math Homework. Download our free learning tools apps and test prep books. Right Triangle Similarity Acute Angle Similarity If one of the acute angles of a right triangle is congruent to an acute angle of another right triangle, then by Angle-Angle Similarity the triangles are similar. Leg-Leg Similarity If the lengths of the corresponding legs of two right triangles are proportional, then by Side-Angle-Side Similarity the triangles are similar. Hypotenuse-Leg Similarity If the lengths of the hypotenuse and a leg of a right triangle are proportional to the corresponding parts of another right triangle, then the triangles are similar.

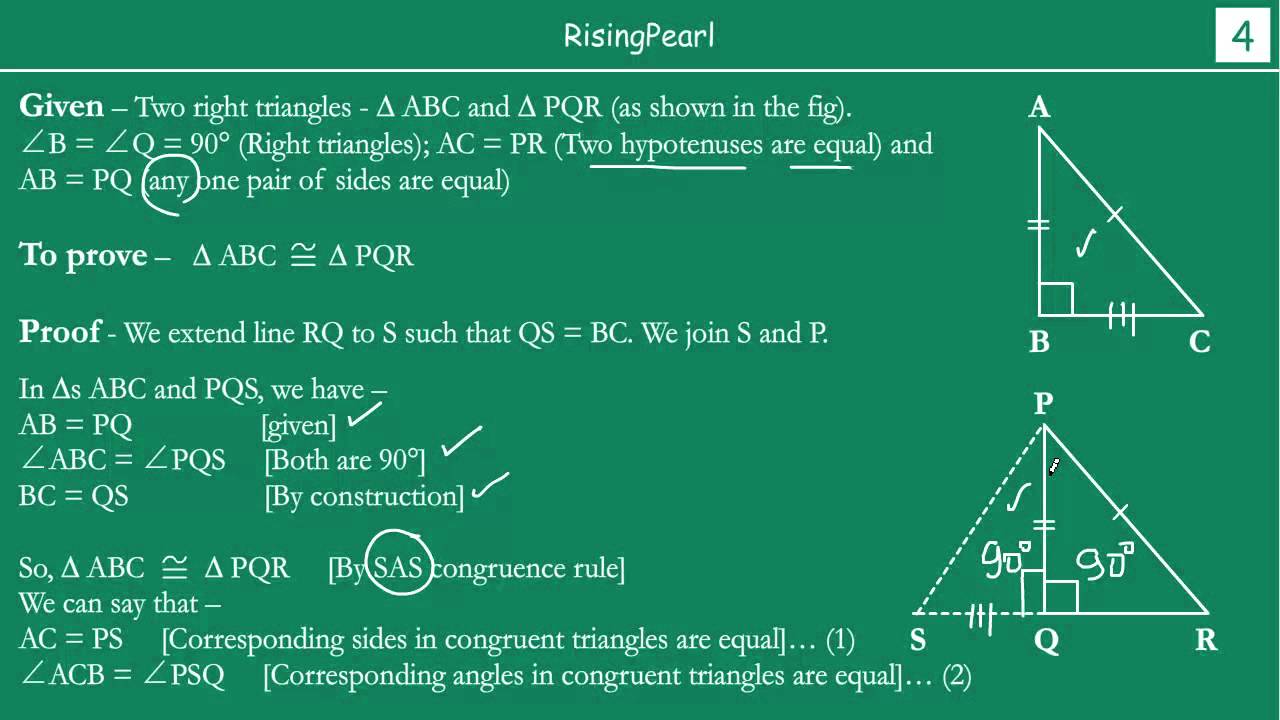
This is a special case of a more general result. To determine if two right triangles satisfy the RHS congruence rule, you need to check if:.

As an avid student of geometry, you likely understand the importance of congruence in shapes and figures. One of the most fundamental rules for determining congruence is the rhs congruence rule. Mastering this rule will open a deeper understanding of triangle congruence and similarity, providing a foundation for more complex geometric proofs and problem solving. In this article, you will explore the rhs congruence rule in detail. Beginning with the definition and formula, you will then see step-by-step how to apply the rule through examples and practice problems.

In Mathematics, a triangle is a closed two-dimensional figure or polygon with the least number of sides. A triangle has three sides and three angles. In this article, let us discuss the important criteria for the similarity of triangles with their theorem and proof and many solved examples. The two triangles are said to be similar triangles , if. Therefore, by using the basic proportionality theorem , we can write. The AA criterion states that if two angles of a triangle are respectively equal to the two angles of another triangle, we can prove that the third angle will also be equal on both the triangles.

Rhs similarity criterion

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Congruent triangles. About About this video Transcript. He also shows that AAA is only good for similarity. For SSA, better to watch next video. Created by Sal Khan. Want to join the conversation? Log in.

B q tiles

Congruency of triangles means that: All corresponding angles are equal. Hire With Us. Fieso Duck. So good questions! It states if in a triangle, all the sides are proportional to the sides of other triangles, then the corresponding angles will always be equal and hence, both triangles are Similar. However, in conjunction with other information, you can sometimes use SSA. Change Language. Explore offer now. We don't need to know that two triangles share a side length to be similar. When working with the RHS congruence rule, it is important to note the following:. Forming triangles using points on a square. Class 10 Math Formulas Class We're not saying that they're actually congruent.

Two triangles are said to be congruent if their corresponding sides and corresponding angles are also congruent.

Enlargements and other transformations in coordinate geometry. Campus Experiences. And that is equal to AC over XZ. So for example, if this is 30 degrees, this angle is 90 degrees, and this angle right over here is 60 degrees. Section 3 can then introduce similarity in terms of enlargement transformations. Create Improvement. For SAS for congruency, we said that the sides actually had to be congruent. Lesson Plan 1. Given that 1 Angstromunit is 10 metres, express this scale as the ratio of two numbers. SSA establishes congruency if the given sides are congruent that is, the same length.