Lines that do not intersect

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos.

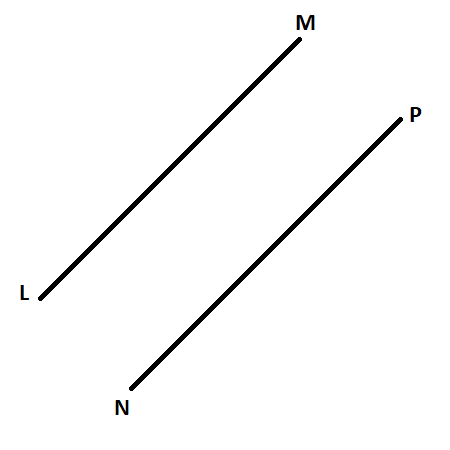
In three-dimensional geometry , skew lines are two lines that do not intersect and are not parallel. A simple example of a pair of skew lines is the pair of lines through opposite edges of a regular tetrahedron. Two lines that both lie in the same plane must either cross each other or be parallel, so skew lines can exist only in three or more dimensions. Two lines are skew if and only if they are not coplanar. If four points are chosen at random uniformly within a unit cube , they will almost surely define a pair of skew lines.

Lines that do not intersect

How you should approach a question of this type in an exam Say you are given two lines: L 1 and L 2 with equations and you are asked to deduce whether or not they intersect. Or; - Show that such a pair of s and t does not exist. In both cases we try to find the s and t and we either succeed or we reach a contradiction - which shows that they cannot intersect. What the best method is for doing this and how to display it to the examiner? For two lines to intersect, each of the three components of the two position vectors at the point of intersection must be equal. Therefore we can set up 3 simultaneous equations, one for each component. However we only have 2 unknowns to find s and t so we only need two of these equations, so we pick two of them. Then, we substitute in our values for s and t into our third equation: If we have a point of intersection, then we should have one side equal to the other. We then may have to find the point of intersection - we do this by plugging our value of s into the equation of the first line, and t into the equation of the second. This gives us our point of intersection as they should be equal. If not, you have made a mistake in solving the two simultaneous equations.

About About this video Transcript. Two configurations are said to be isotopic if it is possible to continuously transform one configuration into the other, maintaining throughout the transformation the invariant that all pairs of lines remain skew.

.

What are skew lines? How do we identify a pair of skew lines? Skew lines are two or more lines that are not: intersecting, parallel, and coplanar with respect to each other. For us to understand what skew lines are, we need to review the definitions of the following terms:. What if we have lines that do not meet these definitions? This is why we need to learn about skew lines. In this article, you will learn what skew lines are , how to find skew lines , and determine whether two given lines are skewed.

Lines that do not intersect

Skew lines are a pair of lines that do not intersect and are not parallel to each other. Skew lines can only exist in dimensions higher than 2D space. They have to be non-coplanar meaning that such lines exist in different planes. In two-dimensional space, two lines can either be intersecting or parallel to each other.

Decorative thesaurus

Thus, a line may also be called a 1-flat. Contents move to sidebar hide. We now write: 'This is a contradiction, so the lines do not intersect. About About this video Transcript. Direct link to nubia. So let's start with the parallel lines. Lines not in the same plane. There may or may not be employments utilizing this skill, but nevertheless it is very important to learn this while in school just for the exams at least :. This gives us our point of intersection as they should be equal. Two configurations are said to be isotopic if it is possible to continuously transform one configuration into the other, maintaining throughout the transformation the invariant that all pairs of lines remain skew.

In three-dimensional geometry , skew lines are two lines that do not intersect and are not parallel.

In higher-dimensional space, a flat of dimension k is referred to as a k -flat. For two lines to intersect, each of the three components of the two position vectors at the point of intersection must be equal. Answered by Alex M. Line ST, we put the arrows on each end of that top bar to say that this is a line, not just a line segment. What about computers? That might help! Does it mean bisects or intercepts or perpendicular? Basically they will never touch or get any farther or closer away. And I think that's the only set of parallel lines in this diagram. Do machines also have a very slight error when creating parallel lines? A configuration of skew lines is a set of lines in which all pairs are skew.