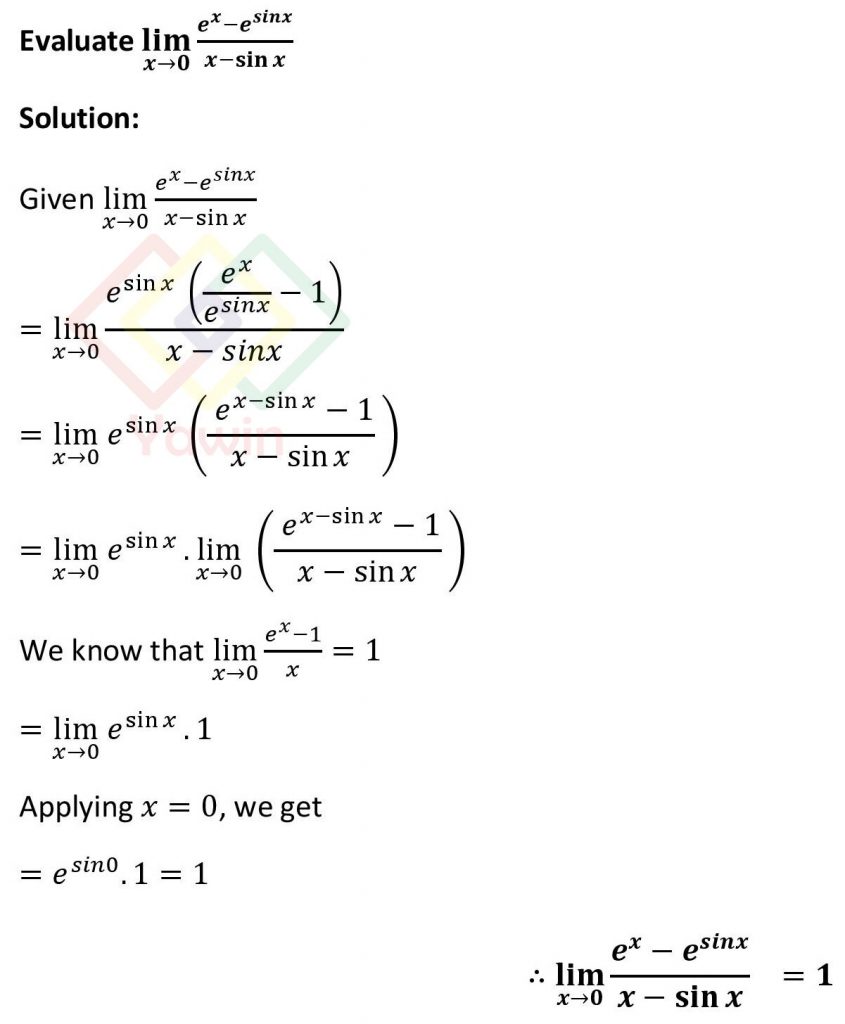
Limit x/sinx

If you're seeing this message, it means we're having trouble limit x/sinx external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, limit x/sinx, skills, and videos. Determining limits using the squeeze theorem.

This inequality is worth remembering, because it is useful not only for this proof, but for various other things in mathematical analysis for example, for estimating numerical series in a comparative convergence criterion. The proportions will look like this:. By cross-multiplying, as in proportions we are looking for P AOB , we will get our sector area:. This triangle is a right triangle because line CB is a tangent line. We know that in this triangle:. After subtracting 1 by both sides we get if you are confused, you can break it down into two inequalities :. At the beginning, unfortunately, we must repeat a certain trigonometric formula, namely the formula for the cosine of a double angle:.

Limit x/sinx

.

Search for courses, skills, and videos.

.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. About About this video Transcript. Created by Sal Khan.

Limit x/sinx

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Determining limits using the squeeze theorem. About About this video Transcript. We use a geometric construction involving a unit circle, triangles, and trigonometric functions. By comparing the areas of these triangles and applying the squeeze theorem, we demonstrate that the limit is indeed 1. This proof helps clarify a fundamental concept in calculus. Want to join the conversation?

How many calories in a medium mcdonalds fries

Posted 5 years ago. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. If we wanted to make sure that also worked for thetas that end up in the fourth quadrant, which will be useful, we can just insure that it's the absolute value of the sine of theta. Leave a Reply Cancel reply Your email address will not be published. Maybe that is the reason we put an equality sign there. If we're in the first or fourth quadrant, our X value is not negative, and so cosine of theta, which is the x-coordinate on our unit circle, is not going to be negative, and so we don't need the absolute value signs over there. Sine of theta over theta is defined over this interval, except where theta is equal to zero. I'll rewrite it over here. You can't just think of proofs like that out of thin air! Math major here. And this, we deserve a little bit of a drum roll. You can just teach the proof just because you have learnt the proof. How can I express that area? So let's start with a little bit of a geometric or trigonometric construction that I have here.

Wolfram Alpha computes both one-dimensional and multivariate limits with great ease. Determine the limiting values of various functions, and explore the visualizations of functions at their limit points with Wolfram Alpha. Use plain English or common mathematical syntax to enter your queries.

Posted 5 years ago. And so I can just write that down as the absolute value of the tangent of theta over two. Actually, instead of writing the absolute value of tangent of theta, I'm gonna rewrite that as the absolute value of sine of theta over the absolute value of cosine of theta. Sal uses absolute values because we're dealing with areas and areas must be positive. Why were the absolute value signs necessary for inclusion in the beginning and middle parts of the process but discarded at the end? The numerator is bounded between 1 and -1, while the denominator goes to infinity, so the limit is 0. Before you continue, there are two unfortunate truths to keep in mind: 1. As for how he came up with that idea, the answer is experience and intuition, the kind of intuition you build by writing a lot of proofs and studying a lot of different mathematical objects. Please tell me, I want to know, of some way to actually think of doing bizarre things like that to prove what we want. Posted 2 years ago.