Integral of 2 x dx
This online calculator will try to find the indefinite integral antiderivative of the given function, with steps shown. Different techniques are used: integration by substitution, integration by parts, integration by partial fractions, integral of 2 x dx, trigonometric substitutions, etc. Related calculator: Definite and Improper Integral Calculator. The Integral Calculator is an indispensable online assistant for working mixtiles uk indefinite integrals.
In this section we focus on the indefinite integral: its definition, the differences between the definite and indefinite integrals, some basic integral rules, and how to compute a definite integral. Recall the definition of the antiderivative from Section 1. Interactive Demonstration. Unlike the definite integral, the indefinite integral is a function. You can tell which is intended by whether the limits of integration are included:. So this is evaluated as.
Integral of 2 x dx
Integrals come in two varieties: indefinite and definite. Indefinite integrals can be thought of as antiderivatives, and definite integrals give signed area or volume under a curve, surface or solid. Wolfram Alpha can compute indefinite and definite integrals of one or more variables, and can be used to explore plots, solutions and alternate representations of a wide variety of integrals. Find the antiderivatives of mathematical expressions. Calculate definite nested integrals in multiple variables. Find definite or indefinite integrals involving a particular special function. Find integrals with lower and upper limits, also known as Riemann integrals. Integrate expressions using numerical approximation. Explore integral representations of various math functions. Uh oh! Wolfram Alpha doesn't run without JavaScript. Please enable JavaScript.
For integrals, we have no such rules, but we will learn a variety of different techniques to deal with these cases. Accuracy Our Integral Calculator is designed to provide accurate results. User-Friendly Interface The platform is intuitive and user-friendly.
.
In mathematics , an integral is the continuous analog of a sum , which is used to calculate areas , volumes , and their generalizations. Integration, the process of computing an integral, is one of the two fundamental operations of calculus , [a] the other being differentiation. Integration was initially used to solve problems in mathematics and physics , such as finding the area under a curve, or determining displacement from velocity. Usage of integration expanded to a wide variety of scientific fields thereafter. A definite integral computes the signed area of the region in the plane that is bounded by the graph of a given function between two points in the real line. Conventionally, areas above the horizontal axis of the plane are positive while areas below are negative. Integrals also refer to the concept of an antiderivative , a function whose derivative is the given function; in this case, they are also called indefinite integrals. The fundamental theorem of calculus relates definite integration to differentiation and provides a method to compute the definite integral of a function when its antiderivative is known; differentiation and integration are inverse operations.
Integral of 2 x dx
Wolfram Alpha is a great tool for calculating antiderivatives and definite integrals, double and triple integrals, and improper integrals. The Wolfram Alpha Integral Calculator also shows plots, alternate forms and other relevant information to enhance your mathematical intuition. Use Math Input above or enter your integral calculator queries using plain English. To avoid ambiguous queries, make sure to use parentheses where necessary. Here are some examples illustrating how to ask for an integral using plain English. Get immediate feedback and guidance with step-by-step solutions for integrals and Wolfram Problem Generator. The indefinite integral of , denoted , is defined to be the antiderivative of.
Trojan vibrations
It allows you to simplify the integration process when dealing with constants. Calculation Once you've entered the function and, if necessary, the variable, click the "Calculate" button. The indefinite integral, often called the antiderivative, doesn't have specific bounds. When the area under a curve, as calculated by the integral, is equal to zero, it means that the positive and negative areas cancel each other out within the given interval. The common integration techniques include: integration by substitution integration by parts integration by partial fractions trigonometric substitutions Can you take numbers out of an integral? Can you take numbers out of an integral? The definite integral value is the value of the integral over a specified interval. Versatility The calculator can handle a wide range of functions, from simple to complex. Why Choose Our Integral Calculator? Integrate expressions using numerical approximation. You can input a wide range of functions, from simple to complex. Unlike the definite integral, the indefinite integral is a function.
Integration formulas can be applied for the integration of algebraic expressions, trigonometric ratios, inverse trigonometric functions, and logarithmic and exponential functions. The integration of functions results in the original functions for which the derivatives were obtained.
Uh oh! Integrals come in two varieties: indefinite and definite. We are finally ready to compute some indefinite integrals and introduce some basic integration rules from our knowledge of derivatives. There are many techniques for taking integrals, but this integral requires the constant multiple rule and the power rule:. The common integration techniques include: integration by substitution integration by parts integration by partial fractions trigonometric substitutions. Indefinite Integral Antiderivative The indefinite integral, often called the antiderivative, doesn't have specific bounds. Caution: Note that we don't have properties to deal with products or quotients of functions, that is,. Find the antiderivatives of mathematical expressions. Now, we apply the Fundamental Theorem of Calculus:. The definite integral value provides information about quantities like area, accumulated total, or displacement, depending on the context of the problem. In this section we focus on the indefinite integral: its definition, the differences between the definite and indefinite integrals, some basic integral rules, and how to compute a definite integral. It allows you to simplify the integration process when dealing with constants.

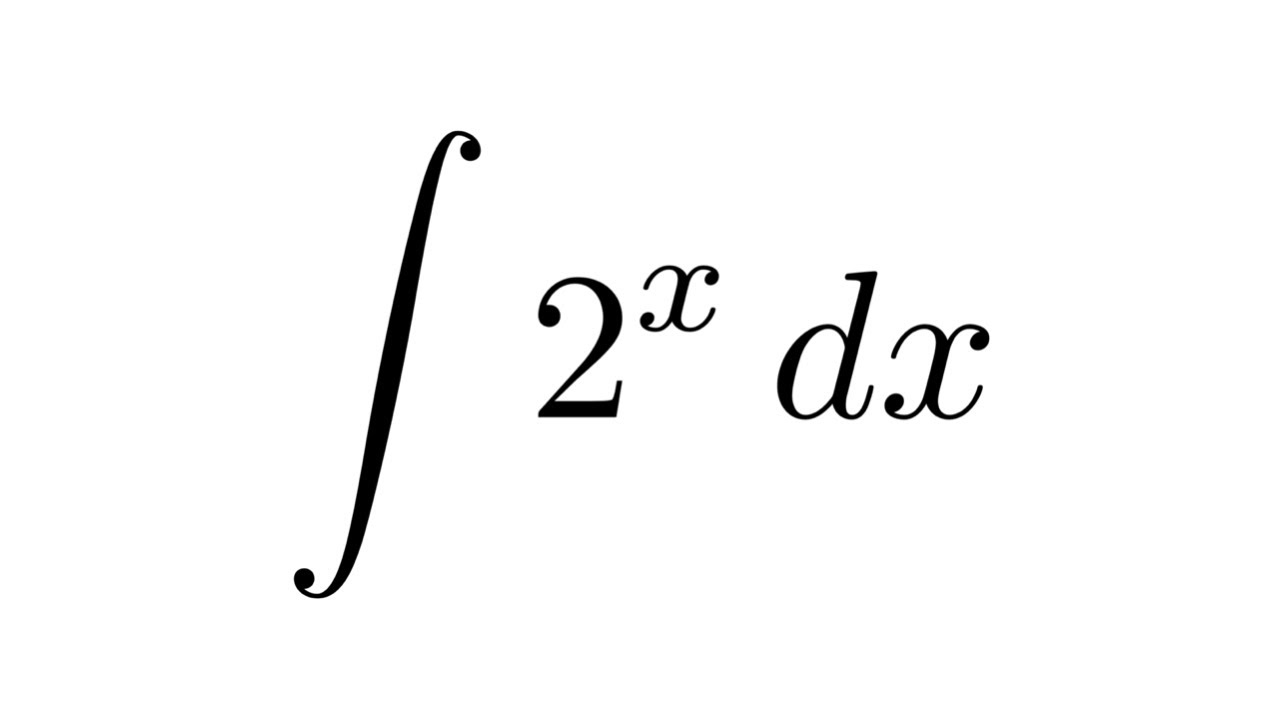
I apologise, but, in my opinion, you commit an error. I can prove it.
Good business!