Formula for sum of ap

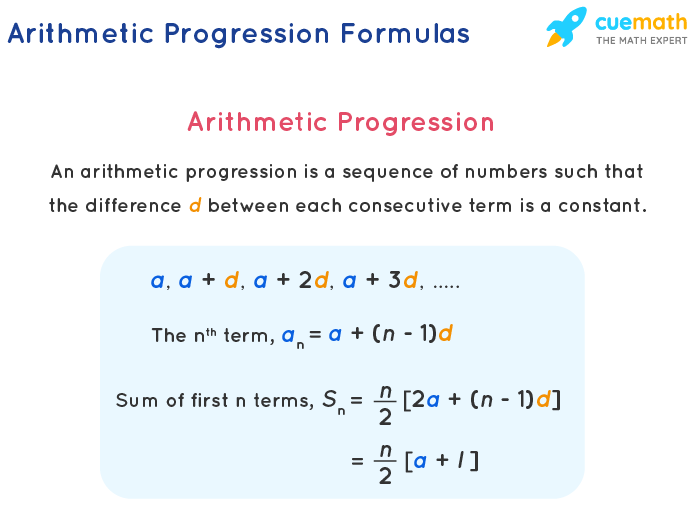
An arithmetic progression AP is a sequence where the differences between every two consecutive terms are the same. For example, the sequence 2, 6, 10, 14, … is an arithmetic progression AP because it follows a pattern where each number is obtained by adding 4 to the previous term. In this article, we will explore the concept of arithmetic progression, the AP formulas to find its n th term, common difference, and the sum formula for sum of ap n terms of an AP. We will solve various examples based on the arithmetic progression formula for a better understanding of the concept.

Sum of n terms in a sequence can be evaluated only if we know the type of sequence it is. Usually, we consider arithmetic progression , while calculating the sum of n number of terms. In this progression, the common difference between each succeeding term and each preceding term is constant. An example of AP is natural numbers, where the common difference is 1. Therefore, to find the sum of natural numbers, we need to know the formula to find it.

Formula for sum of ap

Sum of arithmetic progression formulas maintains a sequence of numbers or a series with the same gap. If we talk about arithmetic progression, it maintains a sequence of numbers or a series of numbers with the same gap or skips between the alternate numbers, and the difference between them remains constant. For example, the sequence 5, 7, 9, 11, 13, 15, … is a progression with a standard difference of two. When there is a finite portion of a progression between the numbers, it is called a limited progression, and sometimes it is just called a progression. The sum of a finite progression is named an arithmetic series. An arithmetic progression AP may be a sequence where the differences between every two consecutive terms are equivalent. There is an opportunity to derive a formula for the nth term in a progression. For instance, the sequence 2, 6, 10, 14, … is a progression AP because it follows a pattern where each number is obtained by adding 4 to its previous term. Arithmetic progression is a progression only when the difference between the series of numbers in the sequence is the same and of the same interval. When the difference obtained by continuously adding a value turns out to be the same, it is known as a common difference. To locate the sum of the arithmetic series to fill the values, Sn, we start with the primary term and successively add on the common difference. We can also start with the nth term and successively subtract the common difference, so,.

Therefore, the sum of the first 20 terms of the series is Square Root Of

An arithmetic progression is a sequence of numbers or variables in which the difference between consecutive terms is the same. There can be an infinite number of terms in an AP. To find the sum of n terms of an AP, we use a formula first founded by Johann Carl Friedrich Gauss in the 19th century. Let us learn all about the sum of n terms of an AP in this article. In the 19th century in Germany, a Math class for grade 10 was going on. The teacher asked her students to sum all the numbers from 1 up to The students were struggling to calculate the sum of all these numbers.

An arithmetic progression is a sequence of numbers or variables in which the difference between consecutive terms is the same. There can be an infinite number of terms in an AP. To find the sum of n terms of an AP, we use a formula first founded by Johann Carl Friedrich Gauss in the 19th century. Let us learn all about the sum of n terms of an AP in this article. In the 19th century in Germany, a Math class for grade 10 was going on. The teacher asked her students to sum all the numbers from 1 up to

Formula for sum of ap

Arithmetic Progression AP is a sequence of numbers in order, in which the difference between any two consecutive numbers is a constant value. It is also called Arithmetic Sequence. For example, the series of natural numbers : 1, 2, 3, 4, 5, 6,… is an Arithmetic Progression, which has a common difference between two successive terms say 1 and 2 equal to 1 2 Even in the case of odd numbers and even numbers, we can see the common difference between two successive terms will be equal to 2. Check: Mathematics for Grade If we observe in our regular lives, we come across Arithmetic progression quite often. For example, Roll numbers of students in a class, days in a week or months in a year. This pattern of series and sequences has been generalized in Maths as progressions. In mathematics, there are three different types of progressions. They are:.

G4s reviews

Ans — In an arithmetic sequence, consecutive terms change by a continuing amount Arithmetic Progression Worksheet. Frequently asked questions. The first term of an arithmetic progression is usually denoted by 'a' or 'a 1 '. We know that to find a term, we can add 'd' to its previous term. Example 1. Therefore, the first term of the Arithmetic Progression is 10 and the common difference of the Arithmetic Progression is 5. In this article, we will learn what is arithmetic progression, summation arithmetic progression , and formulas that can be used to do the calculation as well as solve examples for better understanding. In general, the common difference is the difference between every two successive terms of an AP. The sum of an arithmetic sequence is the sum of all the terms in it. The following table explains the differences between arithmetic and geometric progression :. Sum of n terms in a sequence can be evaluated only if we know the type of sequence it is. Read along to understand the weighted arithmetic mean, its applicability, formula, and advantages. The first term is a and the common difference is 2a.

An arithmetic progression AP is a sequence where the differences between every two consecutive terms are the same.

Want to know more about this Super Coaching? Therefore, the nd term of the given AP 6, 13, 20, 27, 34, To find the sum of n terms of an AP, we use a formula first founded by Johann Carl Friedrich Gauss in the 19th century. Crack K with Unacademy. United States. For example, the sequence 5, 7, 9, 11, 13, 15, … is a progression with a standard difference of two. Multiplication Tables. Hope this article on Sum of Arithmetic Progression formula was informative. Once you ride a taxi you will be charged an initial rate and then a per-mile or per-kilometre charge. Want to build a strong foundation in Math? You will be notified via email once the article is available for improvement. This shows an arithmetic progression that for every kilometre you will be charged a certain fixed constant rate plus the initial rate. Please go through our recently updated Improvement Guidelines before submitting any improvements. Find out more details about an inverse function graph here.