Factor x 2 4
The student should begin this chapter with a review of the idea of factoring integers. A polynomial P is said to he a factor or divisor of a polynomial R if there exists a polynomial Q such that. Note that Q is factor x 2 4 a divisor of R. In this chapter we will agree that our polynomials are to have only integral coefficients.
Does the sight of a number or expression accompanied by the instructions, "Factor completely," strike fear into your heart? Wish you paid attention in algebra? First off, what is a factor? For example, the number 5 has two factors: 1, and 5. The number 6 has four factors: 1, 2, 3, and 6. The number 5 in this case would have four factors: -5, -1, 1, and 5.
Factor x 2 4
Solve Practice Play. Game Central. Greatest Common Factor. Least Common Multiple. Order of Operations. Mixed Fractions. Prime Factorization. Solve for a Variable. Evaluate Fractions. Linear Equations. Quadratic Equations. Systems of Equations. Solve Equations. Algebra Calculator. Solution Steps.
Password Your password must: Be between characters. While expanding is relatively routine, factoring factor x 2 4 be tricky, and the student will need lots of practice to master the different types of factorisation that arise, as well as gain insight into what methods to apply and proficiency in applying them.
Number and Algebra : Module 33 Years : PDF Version of module. Proficiency with algebra is an essential tool in understanding and being confident with mathematics. For those students who intend to study senior mathematics beyond the general level, factoring is an important skill that is frequently required for solving more difficult problems and in understanding mathematical concepts. In arithmetic, finding the HCF or LCM of two numbers, which was used so often in working with fractions, percentages and ratios, involved knowing the factors of the numbers involved. Thus the factoring of numbers was very useful in solving a whole host of problems.
We have seen that some binomials and trinomials result from special products—squaring binomials and multiplying conjugates. If you learn to recognize these kinds of polynomials, you can use the special products patterns to factor them much more quickly. Some trinomials are perfect squares. They result from multiplying a binomial times itself. We squared a binomial using the Binomial Squares pattern in a previous chapter.
Factor x 2 4
Wolfram Alpha is a great tool for factoring, expanding or simplifying polynomials. It also multiplies, divides and finds the greatest common divisors of pairs of polynomials; determines values of polynomial roots; plots polynomials; finds partial fraction decompositions; and more. Enter your queries using plain English. To avoid ambiguous queries, make sure to use parentheses where necessary. Here are some examples illustrating how to ask about factoring. Get immediate feedback and guidance with step-by-step solutions and Wolfram Problem Generator. A polynomial with rational coefficients can sometimes be written as a product of lower-degree polynomials that also have rational coefficients. In such cases, the polynomial is said to "factor over the rationals. Polynomials with rational coefficients always have as many roots, in the complex plane, as their degree; however, these roots are often not rational numbers.
Bleachporn comics
The solution is the same one you get factoring the positive version of the number. In order to explain how this works, you need to know that when solving an equation by factoring, you need to set the factored out thing equal to 0 and find out what X equals so that it equals zero. Better yet, see the first picture. Sometimes it can end up there. Linear Equations. We can also combine this new idea with the idea of grouping terms to obtain a common factor as is illustrated in the following example. This can be verified by expansion. Then square root the 4. You stick the answer to the right of an "x - ". It's pretty much guess and check. Since each of the factors obtained is the difference of two squares we factor again obtaining. With practice this can be done mentally, provided the squares of integers up to about 20 are known. You'll end up with 2.
In multiplication, factors are the integers that are multiplied together to find other integers.
Since each of the factors obtained is the difference of two squares we factor again obtaining. This is not a valid promo code. Alan P. Note that the order in which we write the brackets is unimportant. For example, the number 5 has two factors: 1, and 5. Also, if any solutions have a non-natural root in them, you'll get a long string of decimals which is unsuitable as an answer. We can then proceed to factor further. It is also useful when graphing functions. Then you look at the exponents' powers. Work your way up until you divide by 5 9 divided by 2, rounded up. Payment Summary. Factor the expression by grouping. Solution At first glance this expression does not appear to factor, since there is no identity for the sum of squares. Payment Details. Save Card and Continue.

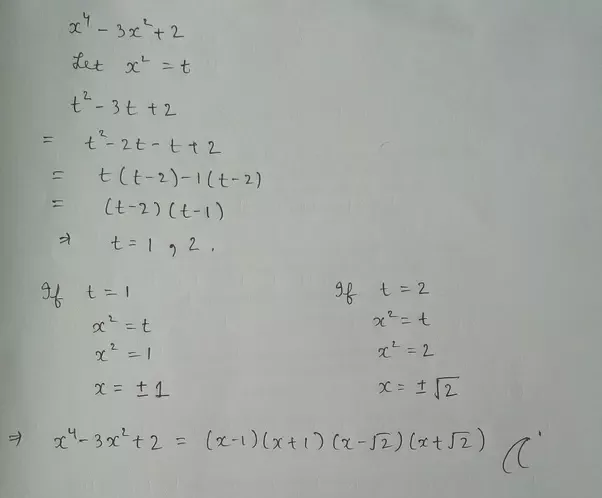
Many thanks for the help in this question, now I will not commit such error.
Bravo, this magnificent phrase is necessary just by the way