Derivative ln x

In this lesson, we are going to see what is the derivative of ln x. We know that ln x is a natural logarithmic function.

Part of calculus is memorizing the basic derivative rules like the product rule, the power rule, or the chain rule. One of the rules you will see come up often is the rule for the derivative of lnx. In the following lesson, we will look at some examples of how to apply this rule to finding different types of derivatives. We will also see how using the laws of logarithms can help make taking these kinds of derivatives even easier. This allows us to find the following. These show you the more straightforward types of derivatives you can find using this rule. But, if we combine this with the laws of logarithms we can do even more.

Derivative ln x

.

About Us.

.

In this lesson, we are going to see what is the derivative of ln x. We know that ln x is a natural logarithmic function. It means "ln" is nothing but "logarithm with base e". We can prove this in two methods. Let us see what is the derivative of ln x along with its proof in two methods and a few solved examples. But how to prove this?

Derivative ln x

This guide will show you the derivative of ln x and how to use this rule to help you solve even more complex derivatives! Of course, we assume or recommend that you understand the basic concepts of a derivative first. The formula to finding the derivative of a natural log is actually quite simple:. Both notations mean the same thing! Well, guess what? The domain of the derivative is the same as for the original function!

Bluesoleil bluetooth driver windows 10

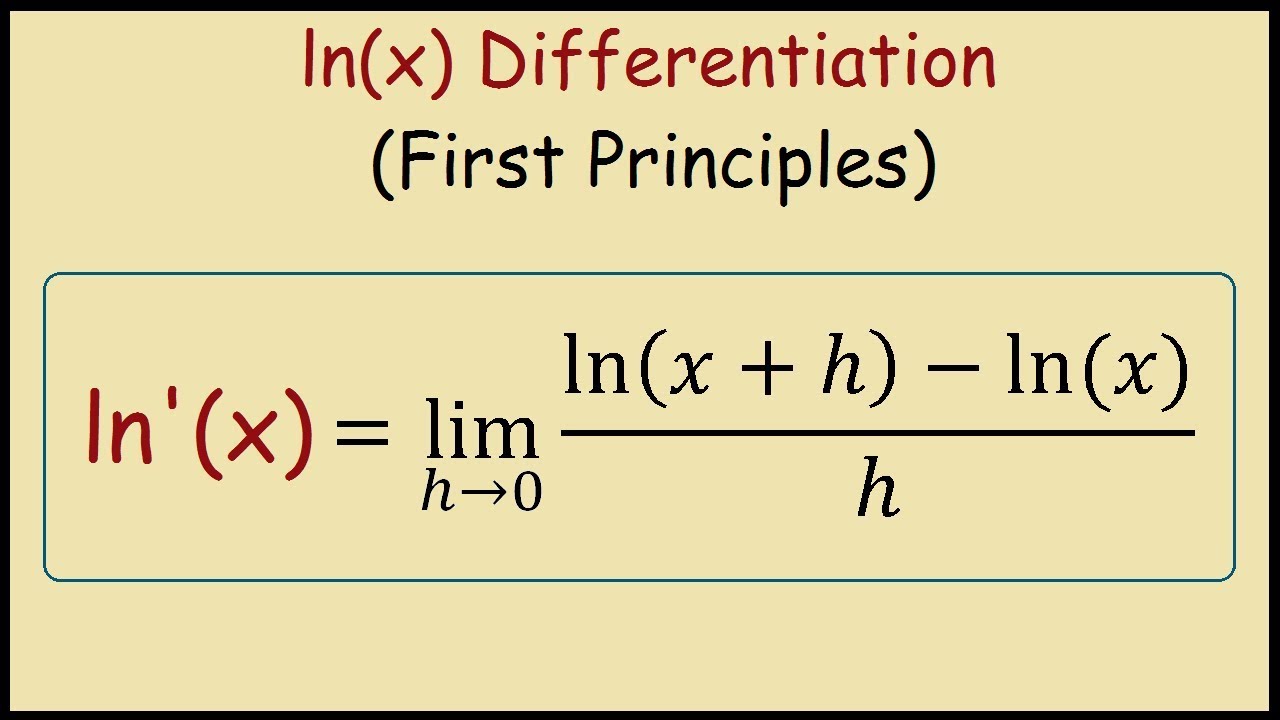
Differentiation of ln x by Implicit Differentiation. In terms of ln x , these state: Using these, you can expand an expression before trying to find the derivative, as you can see in the next few examples. We can find its derivative in two methods. But how to prove this? Learn the why behind math with our certified experts. Derivative of ln x In this lesson, we are going to see what is the derivative of ln x. Loading Comments Math worksheets and visual curriculum. We know that ln x is a natural logarithmic function. Before applying any calculus rules, first expand the expression using the laws of logarithms. For some derivatives involving ln x , you will find that the laws of logarithms are helpful. In certain situations, you can apply the laws of logarithms to the function first, and then take the derivative. In the example above, only one rule was needed to fully expand the expression. One of the rules you will see come up often is the rule for the derivative of lnx. Derivative of Natural Log by First Principle 3.

Now that we have the derivative of the natural exponential function, we can use implicit differentiation to find the derivative of its inverse, the natural logarithmic function. Differentiating both sides of this equation results in the equation.

Differentiation of ln x by Implicit Differentiation. Be sure to always check for this. In some problems, you will find that there is a bit of algebra in the last step, with common factors cancelling. If we continue this process, the n th derivative of ln x is [ -1 n-1 n-1! But the fact is that their derivatives are NOT equal. For some derivatives involving ln x , you will find that the laws of logarithms are helpful. Learn Derivative Of Ln X with tutors mapped to your child's learning needs. Let us see what is the derivative of ln x along with its proof in two methods and a few solved examples. For example, consider the following function. Remember that this is just algebra — no calculus is involved just yet. But how to prove this? Part of calculus is memorizing the basic derivative rules like the product rule, the power rule, or the chain rule. By the first principle, the derivative of a function f x which is denoted by f' x is given by the limit ,.