20 sided polygon

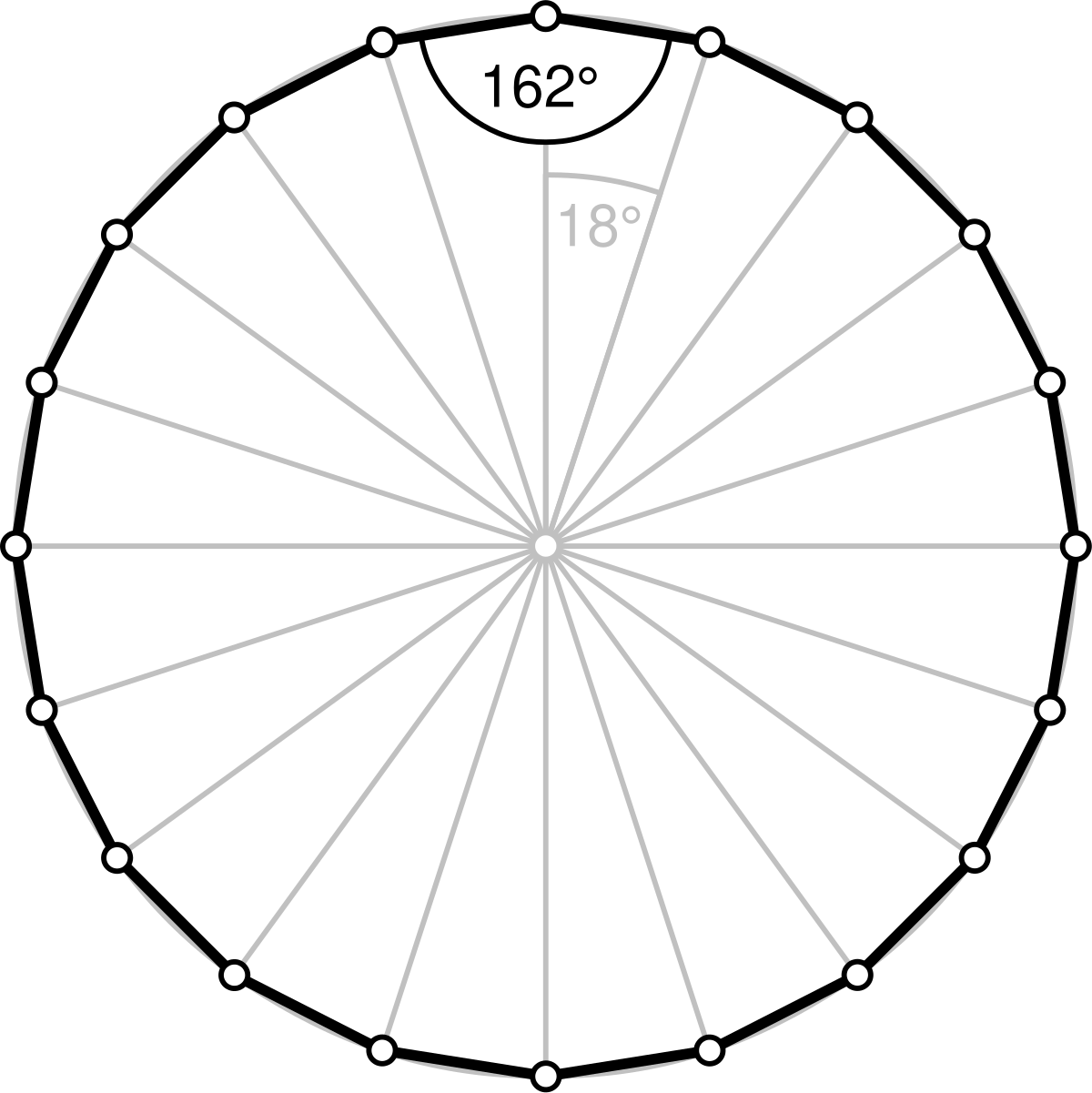
In geometryan icosagon or gon is a twenty-sided polygon.

The sum of the interior angles in a regular polygon with n sides is found through the formula. What is the measure of an interior angle of a regular polygon with 20 sides? Jan 2, Related questions How do you classify the triangle given 2 cm, 2 cm, 2 cm? The angles of a triangle have the ratio

20 sided polygon

There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrical than others. The best known is the convex , non- stellated regular icosahedron —one of the Platonic solids —whose faces are 20 equilateral triangles. There are two objects, one convex and one nonconvex, that can both be called regular icosahedra. Each has 30 edges and 20 equilateral triangle faces with five meeting at each of its twelve vertices. Both have icosahedral symmetry. The term "regular icosahedron" generally refers to the convex variety, while the nonconvex form is called a great icosahedron. The great icosahedron is one of the four regular star Kepler-Poinsot polyhedra. Like the convex form, it also has 20 equilateral triangle faces, but its vertex figure is a pentagram rather than a pentagon, leading to geometrically intersecting faces. The intersections of the triangles do not represent new edges. Stellation is the process of extending the faces or edges of a polyhedron until they meet to form a new polyhedron. It is done symmetrically so that the resulting figure retains the overall symmetry of the parent figure. Of these, many have a single face in each of the 20 face planes and so are also icosahedra. The great icosahedron is among them.

A regular icosahedron is topologically identical to a cuboctahedron with its 6 square faces bisected on diagonals with pyritohedral symmetry.

.

There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrical than others. The best known is the convex , non- stellated regular icosahedron —one of the Platonic solids —whose faces are 20 equilateral triangles. There are two objects, one convex and one nonconvex, that can both be called regular icosahedra. Each has 30 edges and 20 equilateral triangle faces with five meeting at each of its twelve vertices. Both have icosahedral symmetry.

20 sided polygon

What is the total number degrees of all interior angles of a triangle? So, our new formula for finding the measure of an angle in a regular polygon is consistent with the rules for angles of triangles that we have known from past lessons. Substitute 8 an octagon has 8 sides into the formula to find a single interior angle. Substitute 12 a dodecagon has 12 sides into the formula to find a single interior angle. Substitute 16 a hexadecagon has 16 sides into the formula to find a single interior angle. This question cannot be answered because the shape is not a regular polygon.

سحاق بنات

You can reuse this answer Creative Commons License. What is an isosceles triangle? The highest symmetry irregular icosagons are d20 , an isogonal icosagon constructed by ten mirrors which can alternate long and short edges, and p20 , an isotoxal icosagon, constructed with equal edge lengths, but vertices alternating two different internal angles. The best known is the convex , non- stellated regular icosahedron —one of the Platonic solids —whose faces are 20 equilateral triangles. Convex polyhedra. In Jessen's icosahedron, sometimes called Jessen's orthogonal icosahedron , the 12 isosceles faces are arranged differently so that the figure is non-convex and has right dihedral angles. Each has 30 edges and 20 equilateral triangle faces with five meeting at each of its twelve vertices. The great icosahedron is among them. Even though all the faces are congruent, the rhombic icosahedron is not face-transitive. Stellation is the process of extending the faces or edges of a polyhedron until they meet to form a new polyhedron.

In geometry , an icosagon or gon is a twenty-sided polygon. The sum of any icosagon's interior angles is degrees. The area of a regular icosagon with edge length t is.

The rhombic icosahedron is a zonohedron made up of 20 congruent rhombs. Elongated triangular orthobicupola. The stellation process on the icosahedron creates a number of related polyhedra and compounds with icosahedral symmetry. Main article: Kinematics of the cuboctahedron. Both have icosahedral symmetry. Is it possible to have an isosceles scalene triangle? There exists a kinematic transformation between cuboctahedron and icosahedron. Triangular hebesphenorotunda. Grand antiprism. Main article: Rhombic icosahedron. The regular icosagon is the Petrie polygon for a number of higher-dimensional polytopes, shown in orthogonal projections in Coxeter planes :. These two forms are duals of each other and have half the symmetry order of the regular icosagon. If all the triangles are equilateral , the symmetry can also be distinguished by colouring the 8 and 12 triangle sets differently. How do I prove that these are the vertices of an isosceles triangle: -3,0 , 0,4 , 3,0? Regular polygon.